Link to the contact page on my website

Hamilton's stationary action

Currently my main interest is to spread transparent understanding of application of Calculus of Variations in physics, specifically the case of Hamilton's stationary action.

Hamilton's stationary action can be thought of as a machinery with internal moving parts.

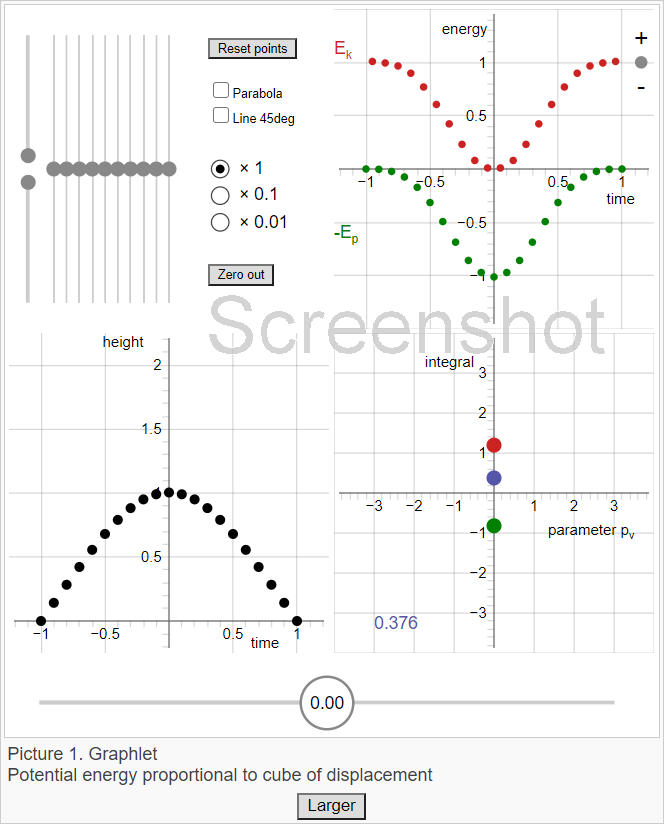
The image below is a screenshot of an interactive diagram that is available on my website. The title of the page is 'Energy-Position equation'.

The purpose of the interactive diagram is to make the internal moving parts transparently visible, similar to how a transparent model of an engine makes the inner workings visible.

In a recent (oktober 8, 2021) answer to a question about the least action concept I took the opportunity to upload diagrams. The diagrams are in the form of animated GIF's, displaying response of energy values to sweeping out variation.

The black dots in the lower left sub-panel mark points along a trial trajectory. Various sliders allow the trial trajectory to be changed in various ways.

The true trajectory is identified as follows:
The true trajectory has the property that the rate of change of kinetic energy matches the rate of change of potential energy over the entire length of the trial trajectory. The two energies are displayed in the upper left sub-panel

The curve for the potential energy has been flipped upside down to emphasize that the slopes of the curves line up: the green curve displays the minus potential energy.

The main slider, at the bottom, does a global variation sweep.

The dots in the lower right sub-panel display the value of the following three integrals:
integral of the kinetic energy (red)
integral of the minus potential energy (green)
Integral of the sum of kinetic energy and minus potential energy (blue)

Motion of the dots in the lower right sub-panel represents motion in response to sweeping out variation. (The variational parameter $p_v$, the horizontal axis, is the value of the main slider.) As the blue dot moves in response to variation, the slope of that motion corresponds to the derivative of Hamilton's action with respect to variation.